Attempts to put probability in QMA. One might be tempted to argue that the probability comes in the assignment of my perceptions to the perceptions of one of the versions; sometimes my perceptions correspond to those of version obs,1, sometimes to those of version obs,2 and so on, with the probability of assignment to version obs,i being |a(i)|². The problem with this conjecture, however, is that when we say ‘my perceptions’, we are implicitly assuming the existence of a single, unique me, separate from the versions. But there is no unique me separate from the versions in QMA; if there are n versions, there are always n equally valid me’s.

Correlation between the coefficients and our perceptions. By looking at a particular case, we can see the problem with probability in QMA from a different and perhaps more compelling perspective. Suppose we consider a two-state system,
with , and suppose we do ten runs, with the observer perceiving the results of every run. There will be 2¹⁰= 1,024 possible outcomes and 1,024 versions of the observer.

Now we know that each of these versions is equally valid (that is, of equal perceptual status) in the sense that (1) each has a valid version of the brain wave function and (2) each version is, within QMA, equally aware (see below). But we know experientially that my perceptions will (almost always) correspond to only one of them, the one with all ten outcomes 1. That is, one version from among all the 1,024 versions is (almost always) singled-out as the one corresponding to my experiential perceptions. Conversely, my perceptions never correspond to one of the 252 versions of the observer that perceives five outcomes 2.

Thus there is a correlation between the coefficients and my perceptions, a correlation which cannot be deduced from the tenets of QMA because perception has no coefficient-dependent or probabilistic characteristics in QMA. Something outside QMA must be responsible for this correlation.

Adding the probability law as an assumption? Suppose we try an interpretation, QMB, which consists of QMA plus the assumption that the probability law holds. This is an allowable interpretation, but it is, in my opinion, unsatisfactory. Why? Because the above argument (principle [P18]) implies there must be some ‘mechanism,’ outside QMA, which is responsible for the correlation, but the simple assumption says nothing about the mechanism. The assumption says, in effect, that the ‘my perception’-coefficient correlation is simply a law of nature.

It seems to me, however, that an interpretation is incomplete if it doesn’t specify the mechanism responsible for the correlation. It seems appropriate to require that a completely satisfactory interpretation explain why our perceptions never correspond to those states with very small amplitudes. Just saying it’s a law of nature leaves the central mystery unexplained. It’s like saying the wavelengths of light emitted by hydrogen atoms follow a regular pattern simply because it’s a law of nature, rather than looking for an underlying explanation.

So I view QMB as only a temporary, unsatisfying interpretation which we use until we understand the cause of the perception-amplitude correlation.

Singling out. The above reasoning shows that there is a correlation between the coefficients and my perceptions. This would almost certainly seem to imply that one version of the observer is singled out as the one whose perceptions correspond to mine. This in turn implies there is a unique me (instead of the many versions of me in QMA). Because no version is singled out in QMA, there must be some non-QMA ‘mechanism’ at work here (see the Probability Law and the Mind-MIND interpretation).


When we perceive the results of an experiment with many potential outcomes, we are consciously aware (however you wish to define that) of one and only one outcome. So we might expect that one and only one version of the observer is aware in quantum physics. But we will show here that that is not the situation in the QMA (no particles, no collapse, no sentient beings) interpretive scheme; all versions are equally aware.

From the point of view of neuroscience or philosophy, what is meant by awareness or consciousness is not so easy to pin down. But we can define it in a general way that is suitable for our purposes here. The state vectors are all that exist in QMA, and so awareness can only correspond to some property—synchronous oscillations of many regions of the brain, for example—of the wave function of the observer’s brain or brain-body.

To see what happens to the observer’s awareness when a measurement results in several simultaneously existing versions of the observer, we consider an experiment on an atomic system with K states, k. There is an apparatus with state vector A and an observer with state vector O. The initial state, before the measurement, with an aware observer, is
We do the measurement in two steps. First we let the apparatus measure and record the results but we cover the readout of the apparatus with an X, so the state is
where the subscript on the observer state indicates that that version is in universe k. Now we have “the observer” look at the reading on the apparatus. We suppose that some versions of the observer are aware and others are not. Then the final state is
But the K states are in separate, non-communicating universes (see Separate Universes), and there can therefore be no coordination between the evolutions of the versions of the observer from the aware state of (16A-3) to either an aware or a not-aware state in (16A-4). The evolution of each version to an aware or not-aware state is individually determined by “chance,” perhaps depending on the way the photons hit the eye in the different versions. This means that, if the not-aware sum is non-zero—if an aware state of the observer is sometimes carried by chance to an unaware state—then by chance (because there is no coordination between versions) there would sometimes be a situation in which no version of the observer was aware at the end.

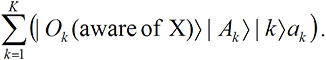
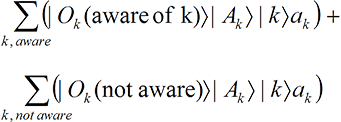
But from our experience of awareness, that is not an allowable outcome; we are always aware of a result. So the only way the time evolution can be made consistent with the at-least-one-aware requirement is to suppose the observer state vector O_k (aware of X) evolves to an aware version, O_k (aware of k), on every branch. All versions are equally aware in QMA, just as aware as the original version of the observer! Thus if “I” perceive one result, there are n – 1 other, equally aware “I”s perceiving the other results.

Comment: There is one way the above argument could fail and that is if the awareness-distinguishing feature is carried by the apparatus or the atomic system. Then the distinguishing feature could be transferred to the observer state vector in the transition from (16A-3) to (16A-4). But a singling-out of this sort amounts to a hidden variable theory (one version ‘physically’ distinguished from the others), and that is forbidden by hypothesis in this section.